-
Seminar COMA Memoriam in honor of the life and work of Jürgen Herzog
Updated on Apr 24, 2024 11:52 AM PDT -
Seminar COMA Working Group: Set Theoretic Complete Intersections
Updated on Feb 15, 2024 10:35 AM PST -
Seminar NAG Colloquium: "Local duality, regularity and completions for stable categories of modular representations"
Updated on May 03, 2024 09:10 AM PDT -
Seminar NAG Donaldson-Thomas Theory Seminar: "4-dimensional Donaldson-Thomas theory”
Updated on May 03, 2024 11:38 AM PDT -
Seminar Commutative Algebra + Algebraic Geometry Seminar: "Classifying numerical semigroups using polyhedral geometry" & "Two bounds on Castelnuovo-Mumford regularity"
Updated on May 03, 2024 09:31 AM PDT -
Seminar COMA Working Group: Quasi-F Splitting
Updated on Feb 09, 2024 01:22 PM PST -
Seminar COMA/NAG Joint Lunch Seminar
Updated on Feb 08, 2024 04:20 PM PST -
Seminar COMA Colloquium: "Local cohomology of subspace arrangements"
Updated on May 03, 2024 08:48 AM PDT -
Seminar COMA Working Group: Syzygies: "Linear syzygies of projective space, by Michael Kemeny"
Updated on May 03, 2024 09:15 AM PDT -
Seminar NAG Categorical Enumerative Invariants Seminar
Updated on Mar 15, 2024 02:01 PM PDT -
Seminar COMA Seminar: "Explicit constructions of short virtual resolutions"
Updated on May 03, 2024 09:17 AM PDT -
Seminar NAG Equivariant Derived Categories Seminar: "Window kernels"
Updated on May 03, 2024 10:21 AM PDT -
Workshop A Celebration for Women in Mathematics (2024) - May 12 Initiative
Organizers: Ini Adinya (University of Ibadan), Nasrin Altafi (Queen's University), Maria-Grazia Ascenzi (University of California Los Angeles), Shanna Dobson (University of California, Riverside), Malena Espanol (Arizona State University), Eleonore Faber (Karl-Franzens-Universität Graz; University of Leeds), Anna Fino (Università di Torino), Adi Glucksam (Northwestern University), Eloísa Grifo (University of Nebraska), Céleste Hogan (Texas Tech University), Ellen Kirkman (Wake Forest University), Kuei-Nuan Lin (Pennsylvania State University), Liangbing Luo (Lehigh University), LEAD Ornella Mattei (San Francisco State University), Claudia Miller (Syracuse University), Julia Plavnik (Indiana University), Claudia Polini (University of Notre Dame), Hema Srinivasan (University of Missouri), Špela Špenko (Université Libre de Bruxelles) "May 12 Initiative" Annual Workshop
"May 12 Initiative" Annual WorkshopThe Simons Laufer Mathematical Sciences Institute (SLMath) celebrates the "May 12 Initiative" with a panel discussion and social event open to all on the topic "Being a Woman in Mathematics". This is a hybrid event taking place on Zoom and in person at SLMath. This event is free and open to worldwide participation.
If you plan to participate online, please connect using this LINK.
Updated on May 03, 2024 01:11 PM PDT -
Seminar COMA Colloquium: "Vanishing Orders of Functions Along Singularities and Ideal Containment Problems in Noetherian Rings"
Updated on Apr 30, 2024 08:11 AM PDT -
Seminar Movie Night: "N is a number: a portrait of Paul Erdős"
Created on Apr 25, 2024 11:14 AM PDT -
MAY-UP Mathematically Advancing Young Undergraduates Program (MAY-UP) 2024
Updated on Apr 26, 2024 08:45 AM PDT -
Seminar COMA Special Topic
Updated on Jan 23, 2024 03:10 PM PST -
Seminar COMA Working Group: Set Theoretic Complete Intersections
Updated on Feb 15, 2024 10:37 AM PST -
Seminar NAG Donaldson-Thomas Theory Seminar
Updated on Feb 13, 2024 01:55 PM PST -
Seminar COMA/NAG Joint Graduate Student Seminar
Updated on Feb 20, 2024 12:44 PM PST -
Seminar NAG Colloquium
Updated on Mar 21, 2024 10:50 AM PDT -
Seminar COMA Working Group: Quasi-F Splitting
Updated on Feb 09, 2024 01:22 PM PST -
Seminar NAG Noncommutative Projective Schemes Seminar
Updated on Feb 22, 2024 10:05 AM PST -
Seminar COMA/NAG Joint Lunch Seminar
Updated on Feb 08, 2024 04:20 PM PST -
Seminar COMA Colloquium
Updated on Jan 23, 2024 12:54 PM PST -
Seminar COMA Working Group: Syzygies
Updated on Feb 15, 2024 10:37 AM PST -
Seminar NAG Categorical Enumerative Invariants Seminar
Updated on Mar 15, 2024 02:01 PM PDT -
Seminar COMA Seminar
Updated on Jan 23, 2024 02:16 PM PST -
Seminar NAG Equivariant Derived Categories Seminar
Updated on Mar 20, 2024 09:39 AM PDT -
Seminar NAG Equivariant Derived Categories Seminar
Updated on Feb 15, 2024 10:38 AM PST -
Seminar COMA/NAG Joint Graduate Student Seminar
Updated on Feb 27, 2024 02:09 PM PST -
Seminar COMA Seminar
Created on Mar 14, 2024 02:07 PM PDT -
Seminar COMA Special Topics
Updated on Mar 14, 2024 02:18 PM PDT -
Seminar COMA Special Topic
Updated on Apr 17, 2024 03:14 PM PDT -
Seminar COMA Working Group: Set Theoretic Complete Intersections
Updated on Feb 15, 2024 10:39 AM PST -
Seminar NAG Colloquium
Updated on Mar 21, 2024 10:50 AM PDT -
Seminar COMA/NAG Joint Graduate Student Seminar
Updated on Feb 20, 2024 12:45 PM PST -
Seminar NAG Donaldson-Thomas Theory Seminar
Updated on Mar 21, 2024 10:49 AM PDT -
Seminar COMA Working Group: Quasi-F Splitting
Updated on Feb 09, 2024 01:22 PM PST -
Seminar NAG Noncommutative Projective Schemes Seminar
Updated on Feb 22, 2024 10:06 AM PST -
Seminar COMA/NAG Joint Lunch Seminar
Updated on Feb 08, 2024 04:20 PM PST -
Seminar COMA Colloquium
Updated on Jan 23, 2024 01:08 PM PST -
Seminar COMA Working Group: Syzygies
Updated on Feb 15, 2024 10:39 AM PST -
Seminar NAG Categorical Enumerative Invariants Seminar
Updated on Mar 15, 2024 02:01 PM PDT -
Seminar COMA Seminar
Updated on Jan 23, 2024 02:16 PM PST -
Seminar NAG Equivariant Derived Categories Seminar
Updated on Mar 20, 2024 09:39 AM PDT -
Seminar NAG Equivariant Derived Categories Seminar
Updated on Feb 15, 2024 10:40 AM PST -
Seminar COMA/NAG Joint Graduate Student Seminar
Updated on Feb 27, 2024 02:09 PM PST -
Seminar COMA Seminar
Updated on Mar 14, 2024 02:18 PM PDT -
Seminar COMA Special Topics
Updated on Mar 14, 2024 02:19 PM PDT -
Workshop Macaulay2, Computational Algebraic Geometry and String Theory
Organizers: David Eisenbud (University of California, Berkeley), Daniel Grayson, Anton Leykin (Georgia Institute of Technology), Andre Lukas (University of Oxford), Devlin Mallory (University of Utah), Liam McAllister (Cornell University), Karl Schwede (University of Utah), Michael Stillman (Cornell University)Updated on Apr 11, 2024 01:36 PM PDT -
Summer Graduate School Séminaire de Mathématiques Supérieures 2024: Flows and Variational Methods in Riemannian and Complex Geometry: Classical and Modern Methods (Montréal, Canada)
Organizers: Vestislav Apostolov (Université du Québec à Montréal), Eleonora Di Nezza (Institut de Mathématiques de Jussieu), Pengfei Guan (McGill University), Spiro Karigiannis (University of Waterloo), Julien Keller (Université du Québec à Montréal), Alina Stancu (Concordia University), Valentino Tosatti (New York University, Courant Institute)
This school will present various developments in Riemannian and Kähler geometry around the notion of curvature seen as a tool to describe and understand the geometry of the objects. The school will give graduate students the opportunity to learn key ideas and techniques of the field, with an emphasis on solidifying foundations in view of potential future research. The first week will be centered around the question of the existence of Kähler metrics with special curvature properties and the famous Yau-Tian-Donaldson conjecture. The second week will focus on geometric flows in Riemannian and complex geometry.
Updated on Mar 18, 2024 02:15 PM PDT -
Summer Research in Mathematics 2024 Summer Research in Mathematics
MSRI/SLMath's Summer Research in Mathematics program provides space, funding, and the opportunity for in-person collaboration to small groups of mathematicians, especially women and gender-expansive individuals, whose ongoing research may have been disproportionately affected by various obstacles including family obligations, professional isolation, or access to funding. Through this effort, MSRI/SLMath aims to mitigate the obstacles faced by these groups, improve the odds of research project completion, and deepen their research experience. The ultimate goal of this program is to enhance the mathematical sciences as a whole by positively affecting the research and careers of all of its participants and assisting their efforts to maintain involvement in the research community.
The ultimate goal of this program is to enhance the mathematical sciences as a whole by positively affecting the research and careers of all of its participants and assisting their efforts to maintain involvement in the research community.
Updated on Sep 14, 2023 09:46 AM PDT -
MSRI-UP MSRI-UP 2024: Mathematical Endocrinology
Organizers: Alexander Diaz-Lopez (Villanova University), Maria Mercedes Franco (Queensborough Community College (CUNY)), Rebecca Garcia (Colorado College), LEAD Candice Price (Smith College), Robin Wilson (Loyola Marymount University)The MSRI-UP summer program is designed to serve a diverse group of undergraduate students who would like to conduct research in the mathematical sciences.
In 2024, MSRI-UP will focus on Mathematical Endocrinology. The research program will be led by Dr. Erica J. Graham, Associate Professor in the Department of Mathematics at Bryn Mawr College.
Updated on Mar 07, 2024 11:11 AM PST -
Summer Graduate School Particle interactive systems: Analysis and computational methods (SLMath)
Organizers: LEAD Irene M. Gamba (University of Texas, Austin), Francois Golse (École Polytechnique), Qin Li (University of Wisconsin-Madison), Chiara Saffirio (Universität Basel)
This summer school will focus on the introductory notions related to the passage of Newtonian and quantum many-body dynamics to kinetic collisional models of Boltzmann flow models arising in statistical sciences in connection to model reductions when continuum macro dynamics arises; and their numerical schemes associated to transport of kinetic processes in classical and data driven mean field dynamics incorporating recent tools from computational kinetics and data science tools. There will be two sets of lectures: “From Newton to Boltzmann to Fluid dynamics”, and “Kinetic collisional theory in mean field regimes: analysis, discrete approximations, and applications”. Each lecture series will be accompanied by a collaboration session, led by the lecturer and teaching assistants. The purpose of the collaboration sessions is to encourage and strengthen higher-level thinking of the materials taught in the lectures and to direct further reading for interested students. Interactive learning activities will be conducted. For example, students will be given problem sets associated with the lectures and will work in small groups to discuss concepts and/or find solutions to assigned problems. The students will also be encouraged to give oral or poster presentations on their solutions or other materials relevant to the course.
Updated on Apr 10, 2024 02:59 PM PDT -
Summer Graduate School Special Geometric Structures and Analysis (St. Mary's College)
Organizers: Costante Bellettini (University College London), LEAD Eleonora Di Nezza (Institut de Mathématiques de Jussieu), Song Sun (University of California, Berkeley) a Calabi-Yau manifold
a Calabi-Yau manifoldThis summer school will serve as an introduction to the SLMath program "Special geometric structures and analysis". There will be two mini-courses: one in Geometric Measure theory and the other in Microlocal Analysis. The aim is to give the basic notions of two subjects also treated during the program.
Updated on Apr 05, 2024 09:40 PM PDT -
African Diaspora Joint Mathematics ADJOINT 2024
ADJOINT is a yearlong program that provides opportunities for U.S. mathematicians – especially those from the African Diaspora – to conduct collaborative research on topics at the forefront of mathematical and statistical research. Participants will spend two weeks taking part in an intensive collaborative summer session at SLMath (formerly MSRI). The two-week summer session for ADJOINT 2024 will take place June 24 to July 5, 2024 in Berkeley, California. Researchers can participate in either of the following ways: (1) joining ADJOINT small groups under the guidance of some of the nation's foremost mathematicians and statisticians to expand their research portfolio into new areas, or (2) applying to Self-ADJOINT as part of an existing or newly-formed independent research group to work on a new or established research project. Throughout the following academic year, the program provides conference and travel support to increase opportunities for collaboration, maximize researcher visibility, and engender a sense of community among participants.
Updated on Apr 10, 2024 10:50 AM PDT -
Summer Graduate School Introduction to Quantum-Safe Cryptography (IBM Zurich)
Organizers: Jonathan Bootle (IBM Zürich Research Laboratory), Luca De Feo (IBM Zürich Research Laboratory)
This two week summer school, jointly organized by SLMath with IBM Zurich, will introduce students to the mathematics and algorithms used in the design and analysis of quantum-safe cryptosystems. Each week will be dedicated to two of the four families of quantum-safe schemes.
Updated on May 01, 2024 08:53 AM PDT -
Summer Graduate School Stochastic Quantization (SLMath)
Organizers: Massimiliano Gubinelli (University of Oxford), Martina Hofmanova (Universität Bielefeld), LEAD Hao Shen (University of Wisconsin-Madison), Lorenzo Zambotti (Sorbonne Université)
This summer school will familiarize students with the basic problems of the mathematical theory of Euclidean quantum fields. The lectures will introduce some of its prominent models and analyze them via the so called “stochastic quantization” methods, involving recently developed stochastic and PDE techniques. This is an area which is highly interdisciplinary combining ideas ranging from the theory of partial differential equations, to stochastic analysis, to mathematical physics. Our goal is to bring together students who are perhaps familiar with some but not all of these subjects and teach them how to integrate these different tools to solve cutting-edge problems of Euclidean quantum field theory.
Updated on Apr 24, 2024 03:23 PM PDT -
Summer Graduate School H-principle (Sendai, Japan)
Organizers: Emmy Murphy (Princeton University), Takashi Tsuboi (RIKEN Interdisciplinary Theoretical and Mathematical Sciences Program) The image of a large sphere isometrically embedded into a small space through a C^1 embedding. (Attributions: E. Bartzos, V. Borrelli, R. Denis, F. Lazarus, D. Rohmer, B. Thibert)
The image of a large sphere isometrically embedded into a small space through a C^1 embedding. (Attributions: E. Bartzos, V. Borrelli, R. Denis, F. Lazarus, D. Rohmer, B. Thibert)This two week summer school, jointly organized by SLMath with RIKEN, will introduce graduate students to the theory of h-principles. After building up the theory from basic smooth topology, we will focus on more recent developments of the theory, particularly applications to symplectic and contact geometry, fluid dynamics, and foliation theory.
h-principles in smooth topology (Emmy Murphy)
Riemannian geometry and applications to fluid dynamics (Dominik Inauen)
Contact and symplectic flexibility (Emmy Murphy)
Foliation theory and diffeomorphism groups (Takashi Tsuboi)Updated on Apr 17, 2024 10:55 AM PDT -
Summer Graduate School Koszul Duality in the Local Langlands Program (St. Mary's College)
Organizers: Clifton Cunningham (University of Calgary), LEAD Sarah Dijols (University of Calgary)
This summer school provides the mathematical background to recognize Koszul duality in representation theory. The school is especially oriented toward applications in the local Langlands program, with an emphasis on real groups. As Koszul duality patterns have been initially observed in the context of Hecke algebras, our school will also introduce the students to Hecke algebras and their categorifications.
Updated on Apr 12, 2024 11:27 PM PDT -
Summer Graduate School Introduction to the Theory of Algebraic Curves (UC Berkeley)
Organizers: Izzet Coskun (University of Illinois, Chicago), Eric Larson (Brown University), LEAD Hannah Larson (University of California, Berkeley), Isabel Vogt (Brown University)
In the last few years, there have been extraordinary developments in many aspects of curve theory. Beginning with many examples in low genus, this summer school will introduce the participants to the background behind these developments in the following areas:
- moduli spaces of stable curves
- Brill–Noether theory
- the extrinsic geometry of the curves in projective space
We will also include an introduction to some open problems at the forefront of these active areas.
Updated on Apr 19, 2024 10:16 AM PDT -
Program Quantum Symmetries Reunion
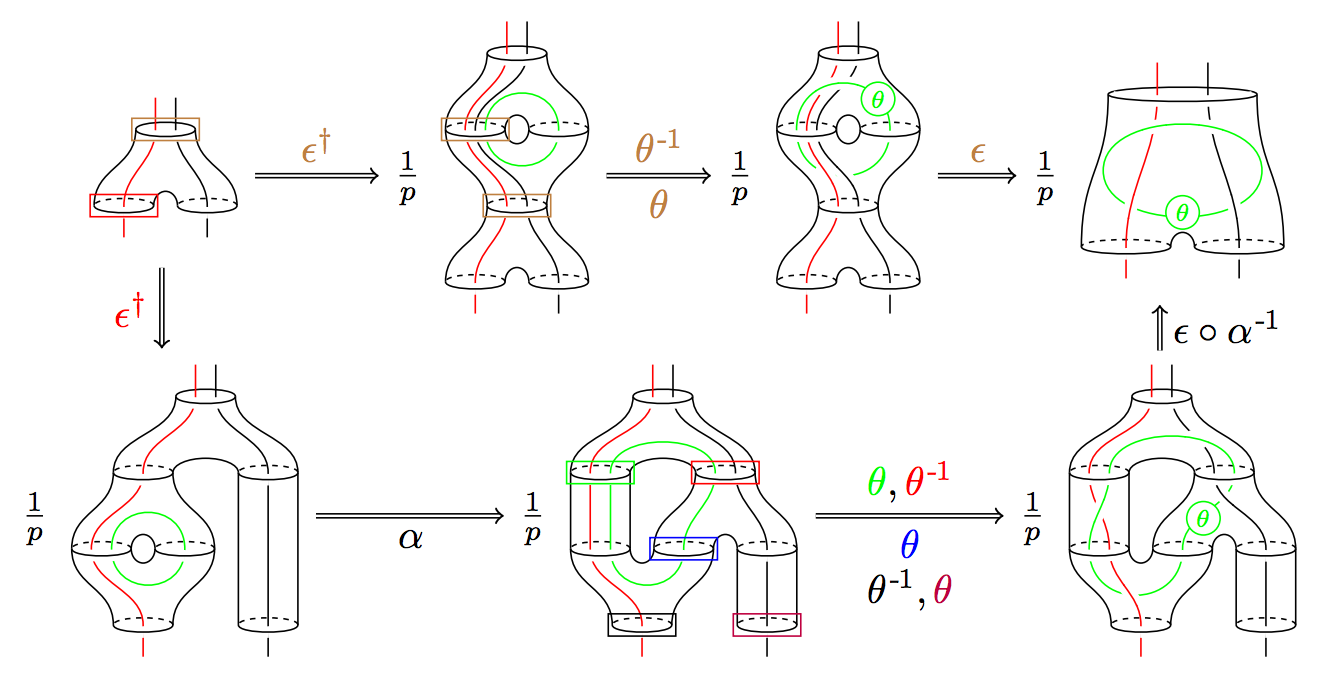 The study of tensor categories involves the interplay of representation theory, combinatorics, number theory, and low dimensional topology (from a string diagram calculation, describing the 3-dimensional bordism 2-category [arXiv:1411.0945]).
The study of tensor categories involves the interplay of representation theory, combinatorics, number theory, and low dimensional topology (from a string diagram calculation, describing the 3-dimensional bordism 2-category [arXiv:1411.0945]).Symmetry, as formalized by group theory, is ubiquitous across mathematics and science. Classical examples include point groups in crystallography, Noether's theorem relating differentiable symmetries and conserved quantities, and the classification of fundamental particles according to irreducible representations of the Poincaré group and the internal symmetry groups of the standard model. However, in some quantum settings, the notion of a group is no longer enough to capture all symmetries. Important motivating examples include Galois-like symmetries of von Neumann algebras, anyonic particles in condensed matter physics, and deformations of universal enveloping algebras. The language of tensor categories provides a unified framework to discuss these notions of quantum symmetry.
Updated on Sep 14, 2023 04:03 AM PDT -
Summer Graduate School Mathematics of General Relativity and Fluids (FORTH, Greece)
Organizers: LEAD Mihalis Dafermos (Princeton University), Grigorios Fournodavlos (University of Crete), Juhi Jang (University of Southern California), Igor Rodnianski (Princeton University) ALCF Visualization and Data Analytics Team; Adam Burrows and the Princeton Supernova Theory Group, Princeton University
ALCF Visualization and Data Analytics Team; Adam Burrows and the Princeton Supernova Theory Group, Princeton UniversityThis summer school will give an accessible introduction to the mathematical study of general relativity, a field which in the past has had barriers to entry due to its interdisciplinary nature, and whose study has been concentrated at specific institutions, to a wider audience of students studying at institutions throughout the U.S., Europe and Greece. Another goal of the summer school will be to demonstrate the common underlying mathematical themes in many problems which traditionally have been studied by separate research communities.
Updated on Mar 19, 2024 04:24 PM PDT -
Summer Graduate School Multigraded and differential graded methods in commutative algebra (St. Mary's College)
Organizers: Michael Brown (Auburn University), Claudia Miller (Syracuse University) Product of projective lines embedded in projective 3-space
Product of projective lines embedded in projective 3-spaceThis summer graduate school focuses on modern homological techniques in commutative algebra, specifically those involving multigraded and differential graded structures. These topics have a long and rich history, but neither is generally covered in graduate courses. Moreover, recent developments have exhibited exciting interplay between the two subjects.
The purpose of the school is to introduce the participants to modern themes on these topics, including Koszul duality for toric varieties and differential graded algebra structures on resolutions. The school will consist of two lectures each day and carefully planned problem sessions designed to reinforce the foundational material, with an emphasis on using computational tools such as the symbolic algebra program Macaulay2.
Updated on Apr 16, 2024 12:43 PM PDT -
Summer Graduate School Analysis of Partial Differential Equations (Okinawa Institute of Science and Technology)
Organizers: Ugur Abdulla (Okinawa Institute of Science and Technology), Gui-Qiang Chen (University of Oxford)This two week summer school, jointly organized by SLMath with OIST, will offer the following two mini-courses:
- Measure-theoretical analysis, divergence-measure fields, and nonlinear PDEs of divergence form
This course will present some recent developments in the theory of divergence-measure fields via measure-theoretic analysis and its applications to the analysis of nonlinear PDEs of conservative form – nonlinear conservation laws. - Perron’s method and Wiener-type criteria in the potential theory of elliptic and parabolic PDEs
This course will present some recent developments precisely characterizing the regularity of the point at ∞ for second order elliptic and parabolic PDEs and broadly extending the role of the Wiener test in classical analysis.
Updated on Apr 19, 2024 12:54 PM PDT - Measure-theoretical analysis, divergence-measure fields, and nonlinear PDEs of divergence form
-
Summer Graduate School Mathematical Spin Glass Theory (Courant, NY)
Organizers: Antonio Auffinger (Northwestern University), Wei-Kuo Chen (University of Minnesota), LEAD Eliran Subag (Weizmann Institute of Science)
While their original aim was to explain the strange behavior of certain magnetic alloys, the study of spin glass models has led to a far-reaching and beautiful physical theory whose techniques have been applied to a myriad of problems in theoretical computer science, statistics, optimization and biology. As many of the physical predictions can be formulated as purely mathematical questions, often extremely hard, about large random systems in high dimensions, in recent decades a new area of research has emerged in probability theory around these problems.
Mathematically, a mean-field spin glass model is a Gaussian process (random function) on the discrete hypercube or the sphere in high dimensions. A fundamental challenge in their analysis is, roughly speaking, to understand the size and structure of their super-level sets as the dimension tends to infinity, which are often studied through smooth objects like the free energy and Gibbs measure whose origin is in statistical physics. The aim of the summer school is to introduce students to landmark results on the latter while emphasizing the techniques and ideas that were developed to obtain them, as well as exposing the students to some recent research topics.
Updated on Apr 19, 2024 03:00 PM PDT -
Program New Frontiers in Curvature: Flows, General Relativity, Minimal Submanifolds, and Symmetry
Organizers: LEAD Ailana Fraser (University of British Columbia), Lan-Hsuan Huang (University of Connecticut), Richard Schoen (Stanford University), LEAD Catherine Searle (Wichita State University), Lu Wang (Yale University), Guofang Wei (University of California, Santa Barbara)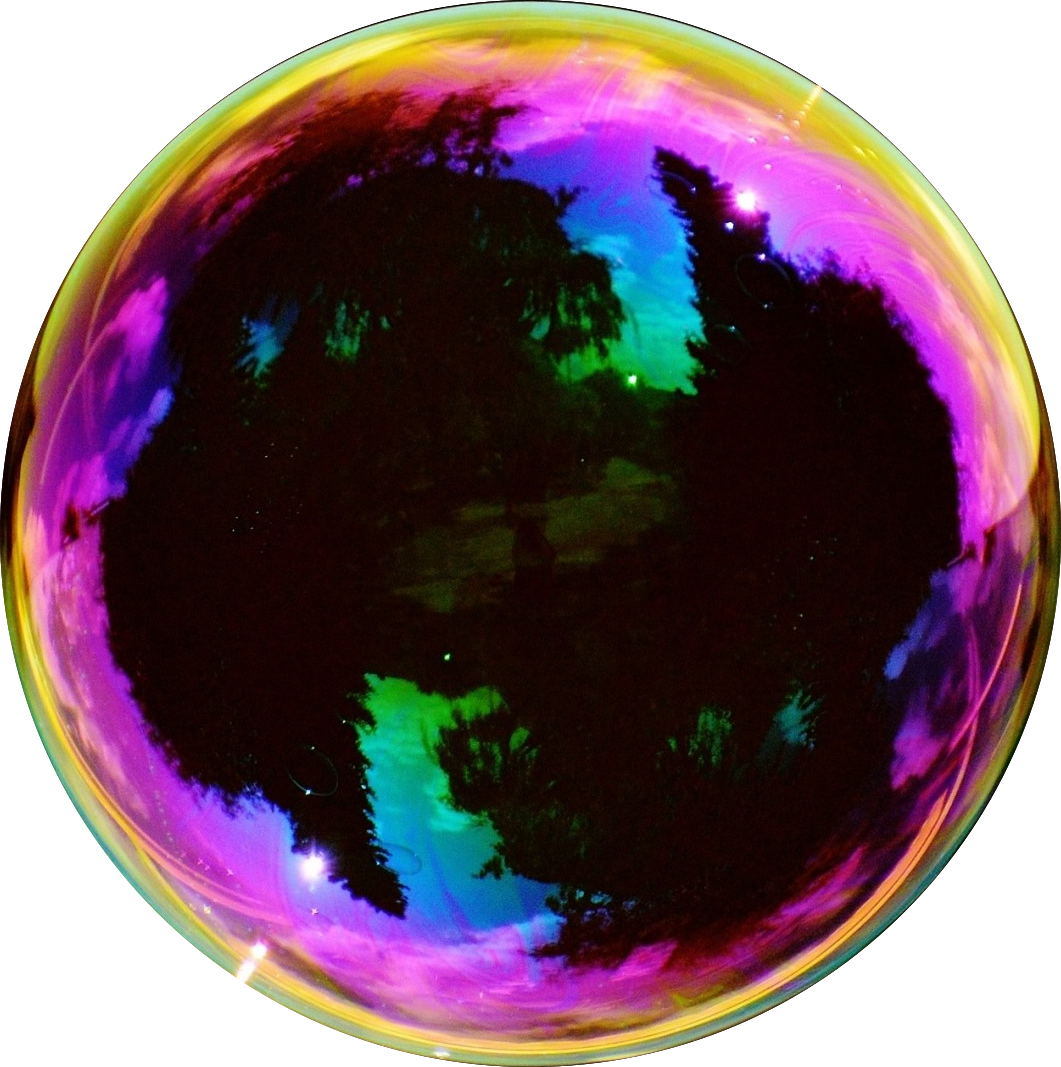 Soap bubble: equilibrium solution of the mean curvature flow and constant curvature surface.
Soap bubble: equilibrium solution of the mean curvature flow and constant curvature surface.Geometry, PDE, and Relativity are subjects that have shown intriguing interactions in the past several decades, while simultaneously diverging, each with an ever growing number of branches. Recently, several major breakthroughs have been made in each of these fields using techniques and ideas from the others.
This program is aimed at connecting various branches of Geometry, PDE, and Relativity and at enhancing collaborations across these disciplines and will include four main topics: Geometric Flows, Geometric problems in Mathematical Relativity, Global Riemannian Geometry, and Minimal Submanifolds. Specifically the program focuses on a central goal, which is to advance our knowledge toward Riemannian (sub)manifolds under geometric conditions, such as curvature lower bounds, by developing techniques in, for example, geometric flows and minimal submanifolds and further fostering new connections.
Updated on Nov 17, 2022 10:10 AM PST -
Program Special Geometric Structures and Analysis
Organizers: Eleonora Di Nezza (Institut de Mathématiques de Jussieu), LEAD Mark Haskins (Duke University), Tristan Riviere (ETH Zurich), Song Sun (University of California, Berkeley), Xuwen Zhu (Northeastern University)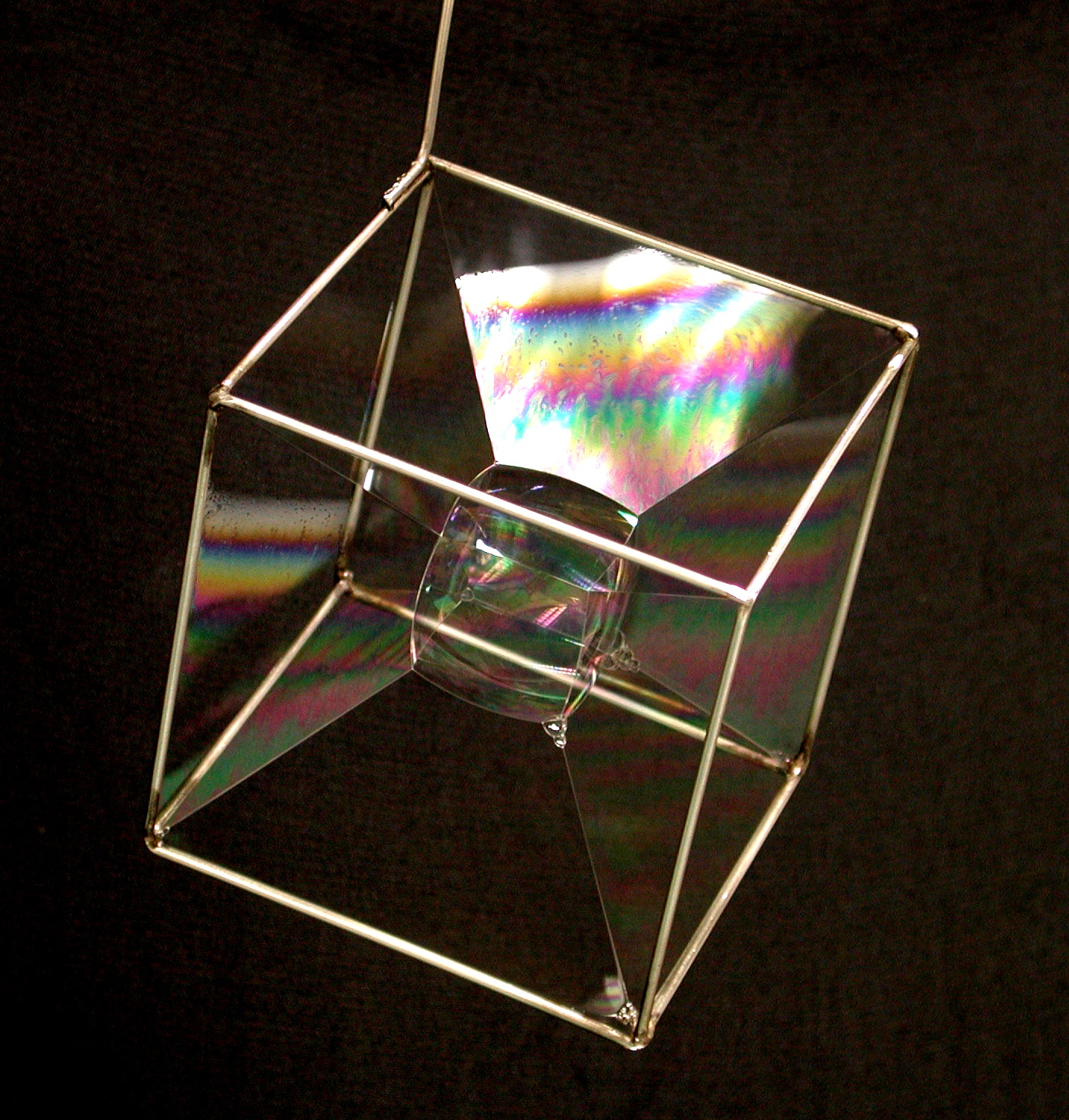 “Plateau’s Memory ” (by A. van der Net): A soap film with singularities
“Plateau’s Memory ” (by A. van der Net): A soap film with singularitiesThis program sits at the intersection between differential geometry and analysis but also connects to several other adjacent mathematical fields and to theoretical physics. Differential geometry aims to answer questions about very regular geometric objects (smooth Riemannian manifolds) using the tools of differential calculus. A fundamental object is the curvature tensor of a Riemannian metric: an algebraically complicated object that involves 2nd partial derivatives of the metric. Many questions in differential geometry can therefore be translated into questions about the existence or properties of the solutions of systems of (often) nonlinear partial differential equations (PDEs). The PDE systems that arise in geometry have historically stimulated the development of powerful new analytic methods. In most cases the nonlinearity of these systems makes ‘closed form’ expressions for a solution impossible: instead more abstract methods must be employed.
Updated on Nov 10, 2022 04:20 PM PST -
Program Complementary Program 2024-25
The Complementary Program has a limited number of memberships that are open to mathematicians whose interests are not closely related to the core programs; special consideration is given to mathematicians who are partners of an invited member of a core program.
Updated on Nov 03, 2023 03:25 PM PDT -
Workshop Connections Workshop: New Frontiers in Curvature & Special Geometric Structures and Analysis
Organizers: Sun-Yung Chang (Princeton University), Lan-Hsuan Huang (University of Connecticut), Chikako Mese (Johns Hopkins University), Ilaria Mondello (Université Paris-Est Créteil Val-de-Marne), LEAD Guofang Wei (University of California, Santa Barbara), LEAD Xuwen Zhu (Northeastern University) Geosurface
GeosurfaceThis three-day workshop will consist of various talks given by prominent female mathematicians on topics of differential geometry and geometric analysis. These will be appropriate for graduate students, post-docs, and researchers in areas related to the two programs. The workshop will also include activities to promote interaction and connection between participants. This workshop is open to all mathematicians.
Updated on Apr 30, 2024 11:01 AM PDT -
Workshop Introductory Workshop: New Frontiers in Curvature
Organizers: Ailana Fraser (University of British Columbia), Karsten Grove (University of Notre Dame), Richard Schoen (Stanford University), Catherine Searle (Wichita State University), LEAD Lu Wang (Yale University) The spatial Schwarzschild space with minimal surface boundary foliated by the inverse mean curvature flow
The spatial Schwarzschild space with minimal surface boundary foliated by the inverse mean curvature flowThis workshop will include introductory lectures on each of the four main topics of the program: geometric flows, geometric problems in mathematical relativity, global Riemannian geometry, and minimal submanifolds. The workshop will also have semi-expository lectures on recent advances and breakthroughs involving interactions between the four main topics. This will set the stage and provide important context for the semester-long program itself.
Updated on Apr 30, 2024 11:03 AM PDT -
Workshop Introductory Workshop: Special Geometric Structures and Analysis
Organizers: Anda Degeratu (Universität Stuttgart), LEAD Eleonora Di Nezza (Institut de Mathématiques de Jussieu), Luca Spolaor (University of California, San Diego), Song Sun (University of California, Berkeley)
This workshop aims to prepare the participants for the main program: Special Geometric Structures and Analysis.
There will be introductory lectures to recent results in geometry and analysis; more precisely in Kähler geometry, special holonomy, microlocal analysis and geometric measure theoryUpdated on Apr 30, 2024 11:05 AM PDT -
Workshop Recent progress on geometric analysis and Riemannian geometry
Organizers: LEAD Lan-Hsuan Huang (University of Connecticut), Andre Neves (University of Chicago), Richard Schoen (Stanford University), LEAD Catherine Searle (Wichita State University), Guofang Wei (University of California, Santa Barbara)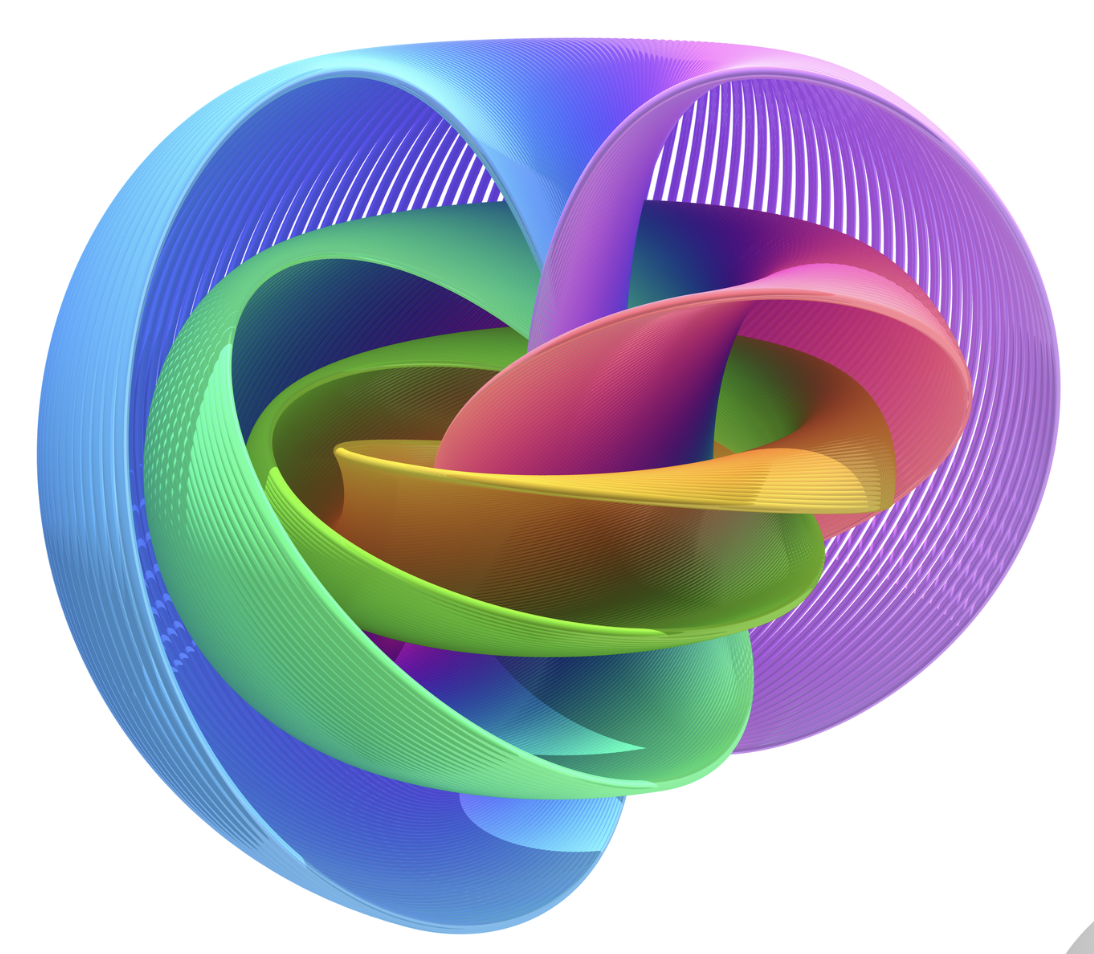 <p>The Hopf fibration of <span class="math-tex">\(S^3 \space by \space S^1\)</span></p>
<p>The Hopf fibration of <span class="math-tex">\(S^3 \space by \space S^1\)</span></p>This workshop will bring together researchers at the frontiers of geometric analysis and Riemannian geometry, with a focus on recent advances on geometric flows, geometric problems in mathematical relativity, global Riemannian geometry, and minimal submanifolds. These areas have shown highly intriguing interactions in recent years and we expect this workshop will provide a unique opportunity to facilitate these emerging links.
Updated on Apr 30, 2024 11:06 AM PDT -
Workshop Geometry and analysis of special structures on manifolds
Organizers: Anna Fino (Università di Torino), Mark Haskins (Duke University), Tristan Riviere (ETH Zurich), Neshan Wickramasekera (University of Cambridge)
The analysis of solutions to nonlinear geometric PDEs with higher-dimensional singular sets has seen some notable recent advances, but many fundamental questions still remain open. This workshop will bring together a wide array of researchers working in differential geometry, gauge theory, nonlinear PDEs, microlocal analysis, the calculus of variations and geometric measure theory, with the goal of describing recent advances, advertising recent technical breakthroughs and forging new connections.
Updated on Apr 30, 2024 11:08 AM PDT -
Workshop Hot Topics: Life after the Telescope Conjecture
Organizers: LEAD Agnes Beaudry (University of Colorado), Michael Hill (University of California, Los Angeles), Vesna Stojanoska (University of Illinois at Urbana-Champaign)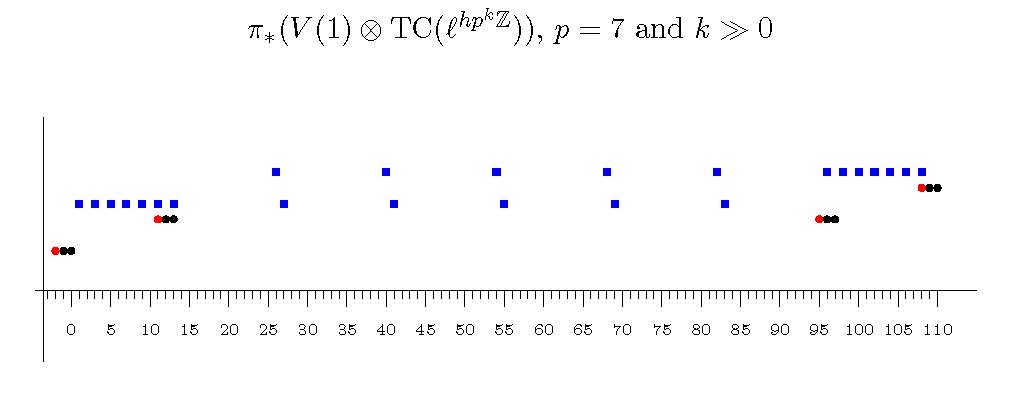
In June 2023, Burklund, Hahn, Levy, and Schlank surprised the homotopy theory community when they announced a disproof of Ravenel's Telescope Conjecture, a fundamental problem of homotopy theory which had been open for 40 years and was believed to be out of reach. The disproof of the Telescope Conjecture combines some of the most exciting recent developments in homotopy theory. This includes fundamental work on red-shift phenomena and descent in algebraic K-theory, trace methods based on a novel approach to topological Hochschild and cyclic homology, ambidexterity in chromatic homotopy theory, and more.
The workshop will explore this amazing body of work, culminating in its synthesis and ingenious application to disprove the Telescope Conjecture.
Updated on Apr 30, 2024 11:09 AM PDT -
Program Probability and Statistics of Discrete Structures
Organizers: Louigi Addario-Berry (McGill University), Christina Goldschmidt (University of Oxford), Po-Ling Loh (University of Cambridge), Gabor Lugosi (ICREA), Dana Randall (Georgia Institute of Technology), LEAD Remco van der Hofstad (Technische Universiteit Eindhoven)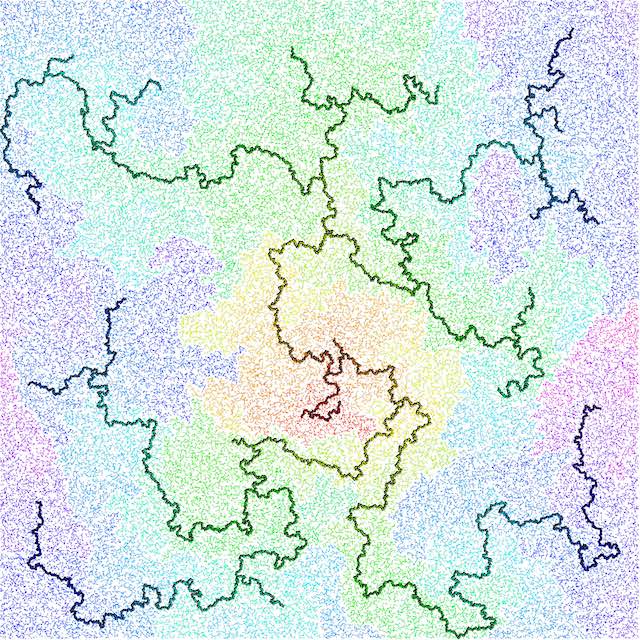 The minimum spanning tree of 100,000 uniformly random points. Colors encode graph distance from the root, which is red. Black points are those whose removal would disconnect at least 5% of the points from the rest.
The minimum spanning tree of 100,000 uniformly random points. Colors encode graph distance from the root, which is red. Black points are those whose removal would disconnect at least 5% of the points from the rest.This program is devoted to the study of the probabilistic and statistical properties of such networks. Central tools include graphon theory for dense graphs, local weak convergence for sparse graphs, and scaling limits for the critical behavior of graphs or stochastic processes on them. The program is aimed at pure and applied mathematicians interested in network problems.
Updated on Feb 29, 2024 02:57 PM PST -
Program Extremal Combinatorics
Organizers: LEAD David Conlon (California Institute of Technology), LEAD Jacob Fox (Stanford University), Penny Haxell (University of Waterloo), Janos Pach (Alfréd Rényi Institute of Mathematics), Maya Stein (Universidad de Chile), Andrew Suk (University of California, San Diego)
Extremal combinatorics concerns itself with problems about how large or small a finite collection of objects can be while satisfying certain conditions. Questions of this type arise naturally across mathematics, so this area has close connections and interactions with a broad array of other fields, including number theory, group theory, model theory, probability, statistical physics, optimization, and theoretical computer science.
Updated on Feb 29, 2024 02:56 PM PST -
Workshop Connections Workshop: Probability and Statistics of Discrete Structures
Organizers: Christina Goldschmidt (University of Oxford), Po-Ling Loh (University of Cambridge), Kavita Ramanan (Brown University), Dana Randall (Georgia Institute of Technology), LEAD Nike Sun (Massachusetts Institute of Technology) AI-generated interpretation of a random network
AI-generated interpretation of a random networkThis two-day workshop will bring together researchers from discrete mathematics, probability theory, theoretical computer science, and statistics to explore topics at their interface. The focus will be on probability and statistics of random discrete structures, as well as their applications, including in computer science and physical systems. The workshop will celebrate academic and gender diversity, bringing together women and men at junior and senior levels of their careers from mathematics, physics, and computer science.
Updated on May 30, 2023 03:32 PM PDT -
Workshop Introductory Workshop: Probability and Statistics of Discrete Structures
Organizers: Louigi Addario-Berry (McGill University), LEAD Shankar Bhamidi (University of North Carolina), Christina Goldschmidt (University of Oxford), Dana Randall (Georgia Institute of Technology), Perla Sousi (University of Cambridge), Remco van der Hofstad (Technische Universiteit Eindhoven) Visualization of a network constructed using simple probabilistic rules, showing the emergence of hubs and other macroscopic network phenomenon. From https://graph-tool.skewed.de
Visualization of a network constructed using simple probabilistic rules, showing the emergence of hubs and other macroscopic network phenomenon. From https://graph-tool.skewed.deNetworks, graph driven algorithms, and dynamics on graphs such as epidemics, random walks and centrality measures all play a major role, both in our daily lives as well as many scientific and engineering disciplines. This introductory workshop will bring together experts and junior researchers in combinatorics, probability, and statistics to share a broad vision of major challenges and objectives, with a primary focus on models of random graphs and their limits, network inference, dynamic processes on networks and algorithms and optimization on random structures.
Updated on May 30, 2023 11:38 AM PDT -
Workshop Connections Workshop: Extremal Combinatorics
Organizers: Julia Böttcher (London School of Economics and Political Science), Anita Liebenau (University of New South Wales), LEAD Maya Stein (Universidad de Chile)
The purpose of this workshop is to bring together promising early-career researchers in extremal combinatorics who are women or from underrepresented minorities so that they can meet with, forge connections with, and be inspired by the leading figures in the area. The workshop will include lectures, time for collaborative research, and an informal panel discussion session among female and minority researchers on career issues.
Updated on Apr 04, 2023 08:43 AM PDT -
Workshop Introductory Workshop - Graph Theory: Extremal, Probabilistic and Structural
Organizers: LEAD Penny Haxell (University of Waterloo), Michael Krivelevich (Tel Aviv University), Alex Scott (University of Oxford)
This workshop will feature leading experts in several major areas of graph theory, including extremal, probabilistic and structural aspects of the field. Introductory lectures will form an important part of the program, providing background and motivation, and aimed at a general mathematical audience. Complementing these, research talks will share exciting recent developments in graph theory.
Updated on Mar 31, 2023 03:48 PM PDT -
Workshop Hot Topics: Interactions between Harmonic Analysis, Homogeneous Dynamics, and Number Theory
Organizers: Dubi Kelmer (Boston College), LEAD Amir Mohammadi (University of California, San Diego), Hong Wang (New York University, Courant Institute)
In recent years techniques from harmonic analysis viz. projection theorems have found striking applications in finitary analysis on homogenous spaces. Such quantitative results have many potential applications to analytic number theory. This workshop will bring together researchers in these areas to further explore these connections.
Updated on Apr 12, 2024 09:01 AM PDT -
Workshop Algebraic and Analytic Methods in Combinatorics
Organizers: Janos Pach (Alfréd Rényi Institute of Mathematics), Andrew Suk (University of California, San Diego), LEAD Yufei Zhao (Massachusetts Institute of Technology) A degree 7 curve passing through 35 points in the plane
A degree 7 curve passing through 35 points in the planeMany exciting breakthroughs in combinatorics involve innovative applications of techniques from a wide range of areas such as harmonic analysis, polynomial and linear algebraic methods, spectral graph theory, and representation theory. This workshop will present recent developments in this area and facilitate discussions of research problems.
Updated on Jul 19, 2023 04:10 PM PDT -
Workshop Detection, Estimation, and Reconstruction in Networks
Organizers: Po-Ling Loh (University of Cambridge), Gabor Lugosi (ICREA), Sofia Olhede (École Polytechnique Fédérale de Lausanne (EPFL)), Roberto Oliveira (Institute of Pure and Applied Mathematics (IMPA)), LEAD Miklos Racz (Northwestern University) Recovering communities in a network.
Recovering communities in a network.In a growing number of applications, one needs to analyze and interpret data coming from massive networks. The statistical problems arising from such applications lead to important mathematical challenges: building novel probabilistic models, understanding the possibilities and limitations for statistical detection and inference, designing efficient algorithms, and understanding the inherent limitations of fast algorithms. The workshop will bring together leading researchers in combinatorial statistics, machine learning, and random graphs in the hope of cross-fertilization of ideas.
Updated on Aug 05, 2023 10:06 AM PDT -
Summer Graduate School Statistical Optimal Transport (St. Mary's College)
Organizers: LEAD Promit Ghosal (Brandeis University), Jonathan Niles-Weed (New York University, Courant Institute), Marcel Nutz (Columbia University)
This summer school offers an exceptional opportunity for participants to delve into the intricate realm of statistical optimal transport theory. This captivating field stands at the crossroads of multiple disciplines, drawing from a rich tapestry of mathematical insights from diverse subjects, including partial differential equations, stochastic analysis, convex geometry, statistics, and machine learning, crafting a vibrant and interdisciplinary landscape. The foremost objective of this summer school is to create a dynamic learning environment that unites students from diverse backgrounds such as PDE theory, probability, or optimal transport.
Updated on Mar 20, 2024 02:04 PM PDT -
Summer Graduate School Noncommutative Algebraic Geometry
Organizers: Pieter Belmans (University of Luxembourg), Lander Hermans (Universiteit Antwerp), Wendy Lowen (Universiteit Antwerpen), Arne Mertens (Universiteit Antwerp), Michel VAN DEN BERGH (Hasselt University), Špela Špenko (Université Libre de Bruxelles)Created on Apr 03, 2024 05:26 PM PDT -
Summer Graduate School Computer Assisted Proofs in Applied Mathematics (SLMath)
Organizers: LEAD Jonathan Jaquette (New Jersey Institute of Technology), Evelyn Sander (George Mason University)
One of the core elements of applied mathematics is mathematical modeling consisting of nonlinear equations such as ODEs, and PDEs. A fundamental difficulty which arises is that most nonlinear models cannot be solved in closed form. Computer assisted proofs are at the forefront of modern mathematics and have led to many important recent mathematical advances. They provide a way of melding analytical techniques with numerical methods, in order to provide rigorous statements for mathematical models that could not be treated by either method alone. In this summer school, students will review standard computational and analytical techniques, learn to combine these techniques with more specialized methods of interval arithmetic, and apply these methods to establish rigorous results in otherwise intractable problems
Updated on Apr 08, 2024 08:55 AM PDT -
Summer Graduate School Geometry and Dynamics in Higher Rank Lie Groups (St. Mary's College)
Organizers: Richard Canary (University of Michigan), Sara Maloni (University of Virginia), Wenyu Pan (University of Toronto), Cagri Sert (University of Zurich), LEAD Tengren Zhang (National University of Singapore) <p>Flats and hyperbolic planes in a higher rank symmetric space</p> Drawn by Steve Trettel.
<p>Flats and hyperbolic planes in a higher rank symmetric space</p> Drawn by Steve Trettel.Lie groups are central objects in modern mathematics; they arise as the automorphism groups of many homogeneous spaces, such as flag manifolds and Riemannian symmetric spaces. Often, one can construct manifolds locally modelled on these homogeneous spaces by taking quotients of their subsets by discrete subgroups of their automorphism groups. Studying such discrete subgroups of Lie groups is an active and growing area of mathematical research. The objective of this summer school is to introduce young researchers to a class of discrete subgroups of Lie groups, called Anosov subgroups.
Updated on Apr 18, 2024 08:43 AM PDT -
Summer Graduate School Topological and Geometric Structures in Low Dimensions (SLMath)
Organizers: LEAD Kenneth Bromberg (University of Utah), Kathryn Mann (Cornell University)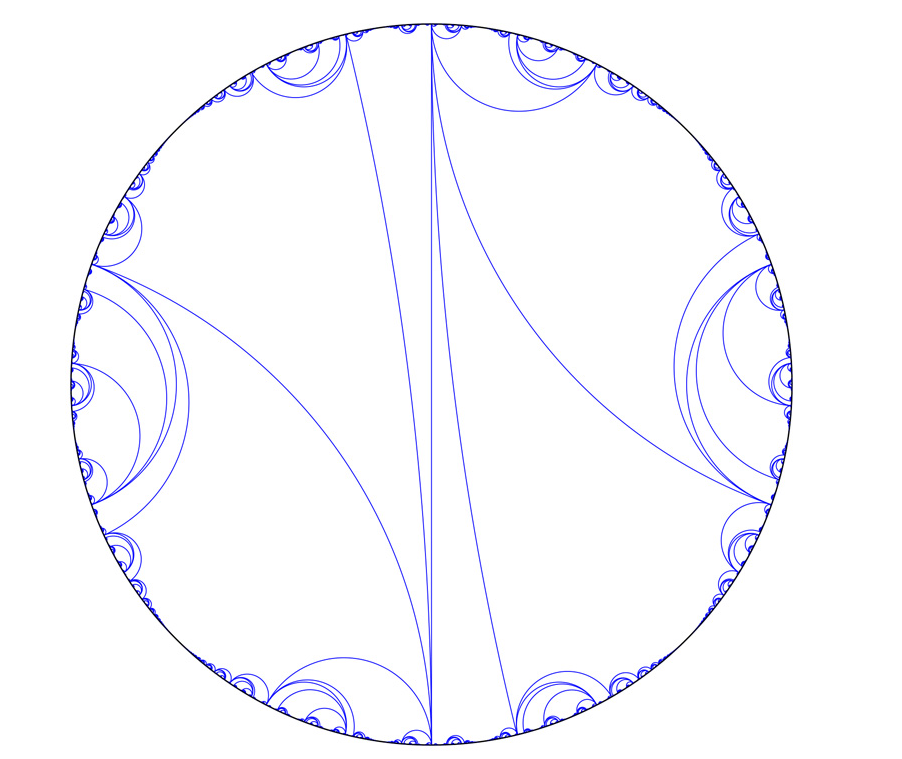 <p>Laminations arise naturally in hyperbolic geometry and (pseudo-) Anosov flows [Image by Jeffrey Brock]</p>
<p>Laminations arise naturally in hyperbolic geometry and (pseudo-) Anosov flows [Image by Jeffrey Brock]</p>This school will serve as an introduction to the SLMath semester “Topological and Geometric Structures in Low-Dimensions”. The school consists of two mini-courses: one on Teichmüller Theory and Hyperbolic 3-Manifolds and the other on Anosov Flows on Geometric 3-Manifolds. Both topics lie at the interface of low-dimensional geometric topology (specifically, surfaces, foliations, and 3-manifolds) and low-dimensional dynamics. The first course will be targeted towards students who have completed the standard first year graduate courses in geometry, topology, and analysis while the second course will geared towards more advanced students who are closer to beginning research. However, we expect that all students will benefit from both courses.
Updated on Mar 21, 2024 09:48 AM PDT -
Program Kinetic Theory: Novel Statistical, Stochastic and Analytical Methods
Organizers: Laurent Desvillettes (Université Paris Cité), Irene M. Gamba (University of Texas, Austin), Francois Golze (École Polytechnique), LEAD Pierre Emmanuel Jabin (Pennsylvania State University), Qin Li (University of Wisconsin-Madison), Chiara Saffirio (Universität Basel), Lexing Ying (Stanford University)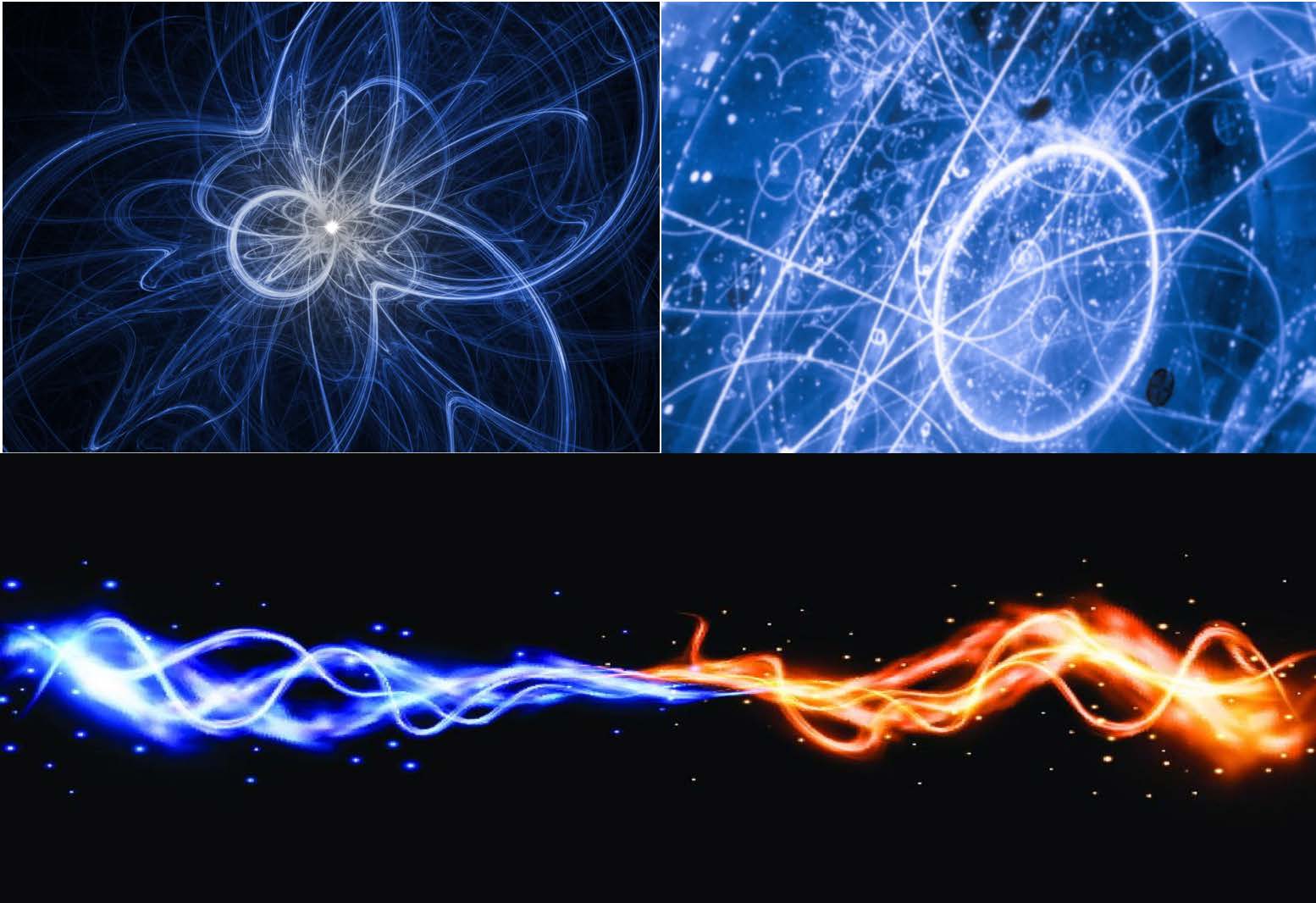 Top: Neutrino interactions and neutrino-atom interactions. Bottom: Collision of two "waves"
Top: Neutrino interactions and neutrino-atom interactions. Bottom: Collision of two "waves"The focus of the proposed program is on so-called kinetic equations, describing the evolution of the of many-particle interacting systems. These models have the form of statistical flows, with their solutions being either a single or multiple point probability density functions or measures, supported in a space of attributes. The attributes are problem-dependent and can be molecular velocity, energy, opinion, wealth, and many others. The flow then predicts the evolution of the probability measure in time, position in space, and the interchanging of the particles' states by the transition probability.
The program will strive to give an overview of the novel mathematical tools used in kinetic theory through a broad range of classical and more recent applications.
Updated on Apr 17, 2024 08:58 AM PDT -
Program Recent Trends in Stochastic Partial Differential Equations
Organizers: Sandra Cerrai (University of Maryland), Yu Gu (University of Maryland), Massimiliano Gubinelli (University of Oxford), Davar Khoshnevisan (University of Utah), Andrea Nahmod (University of Massachusetts, Amherst), Hao Shen (University of Wisconsin-Madison), LEAD Lorenzo Zambotti (Sorbonne Université)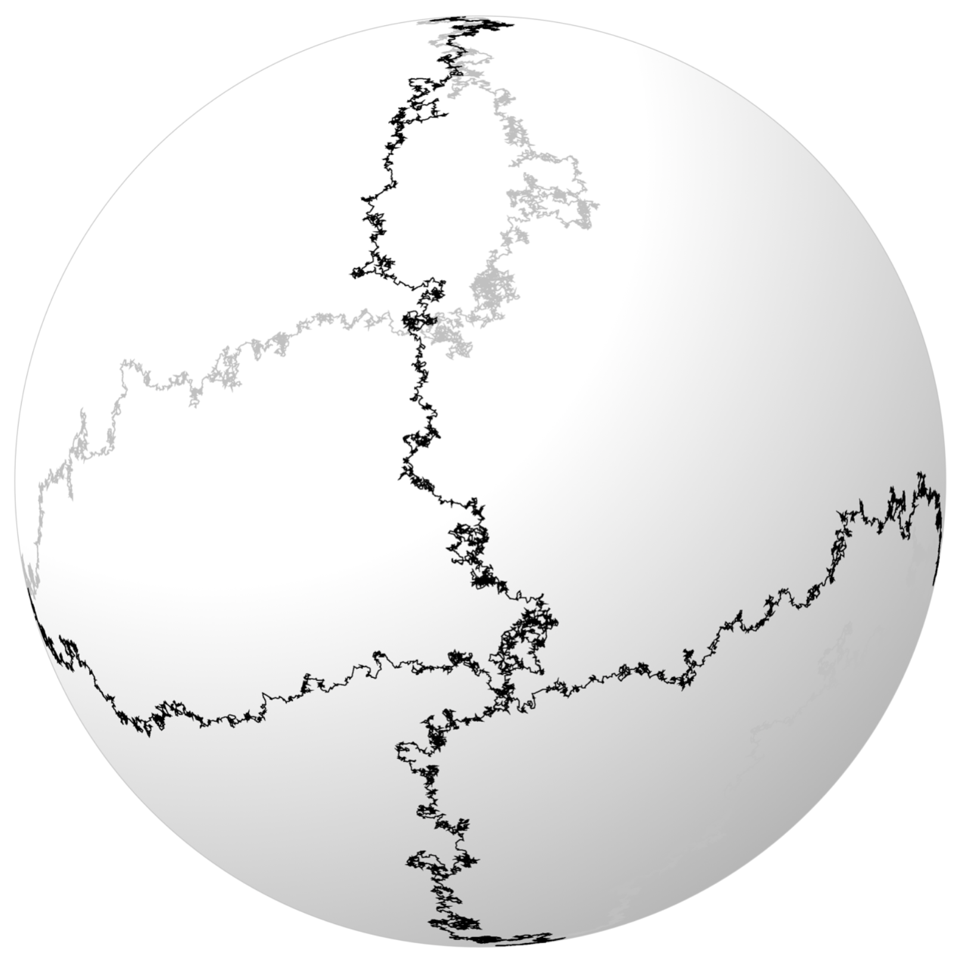 Solution to the geometric stochastic heat equation on the sphere at a fixed time
Solution to the geometric stochastic heat equation on the sphere at a fixed timeThe topic Singular Stochastic Partial Differential Equations (singular SPDE) has rapidly grown to be an active research area at the interface of Stochastic Analysis and PDEs on one hand, and Mathematical Physics on the other hand. During this decade we have witnessed a series of tremendous breakthroughs in the solution theories of SPDEs, universality problems, large-scale asymptotic behaviors of solutions, and foundational relations with quantum field theories and geometry. Many long-standing problems have been resolved via newly developed methods – notably the theories of regularity structures and paracontrolled distributions – and deep connections with other fields are quickly emerging.
It is a natural time to convene a large-scale semester program.
Updated on Apr 17, 2024 10:56 AM PDT -
Program Geometry and Dynamics for Discrete Subgroups of Higher Rank Lie Groups
Organizers: Martin Bridgeman (Boston College), LEAD Richard Canary (University of Michigan), Amir Mohammadi (University of California, San Diego), LEAD Hee Oh (Yale University), Maria Beatrice Pozzetti (Ruprecht-Karls-Universität Heidelberg), Jean-François Quint (CNRS - Université de Montpellier)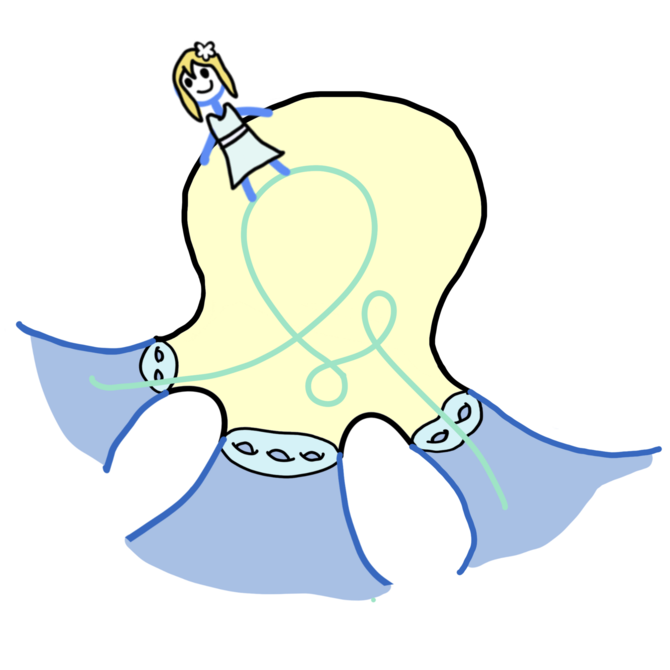 <p>This figure depicts dynamics of flows on convex cocompact hyperbolic 3-manifolds; where the girl is a traveller along a horocycle.</p>
<p>This figure depicts dynamics of flows on convex cocompact hyperbolic 3-manifolds; where the girl is a traveller along a horocycle.</p>This research program will bring together two intellectual communities that have made significant advances in the study of discrete subgroups of higher rank semisimple Lie groups: the homogeneous dynamics community and the community studying geometric structures and Anosov groups.
Updated on Apr 17, 2024 11:08 AM PDT -
Program Topological and Geometric Structures in Low Dimensions
Organizers: Ian Agol (University of California, Berkeley), Kenneth Bromberg (University of Utah), Sebastian Hensel (LMU München), Christopher Leininger (Rice University), Kathryn Mann (Cornell University), LEAD Yair Minsky (Yale University), Rachel Roberts (Washington University in St. Louis)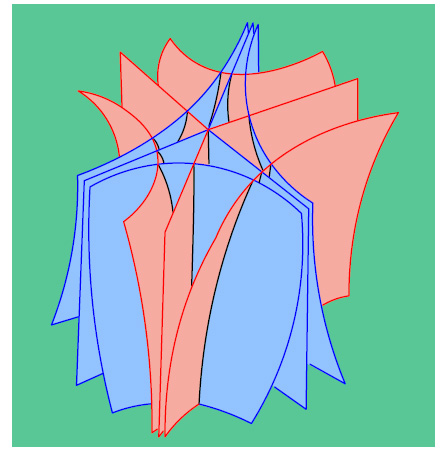 The stable and unstable foliations near a singular orbit of a pseudo- Anosov flow in 3 dimensions. Courtesy Michael Landry.
The stable and unstable foliations near a singular orbit of a pseudo- Anosov flow in 3 dimensions. Courtesy Michael Landry.Low dimensional topology is a meeting place for many objects and ideas from diverse areas of mathematics, including foliation theory, geometry, and smooth and conformal dynamics. For instance, many foliations on 3-manifolds admit transverse flows, connecting (local) leafwise homeomorphisms to flow dynamics and the mapping class groups of the leaves. Leafwise conformal or hyperbolic structures can be approached through Teichmüller theory, and connect again to one-dimensional dynamics through "universal circles" organizing compactifications of all the leaves or of the flow space. Many of these ideas originate in work of Thurston but in recent years have diverged and are ripe for reconnection.
The program will bring together experts in all these fields together with younger researchers, who together can form new connections and open new areas for exploration.Updated on Apr 17, 2024 11:10 AM PDT -
Program New Trends in Tropical Geometry
Organizers: Pierrick Bousseau (University of Georgia), Melody Chan (Brown University), Ilia Itenberg (Institut de Mathématiques de Jussieu - Paris Rive Gauche), Hannah Markwig (Eberhard-Karls-Universität Tübingen), LEAD Kris Shaw (University of Oslo) Tropical surfaces. Images courtesy of Lars Allermann.
Tropical surfaces. Images courtesy of Lars Allermann.Tropical geometry can be viewed as a degenerate version of algebraic geometry,where the role of algebraic varieties is played by certain polyhedral complexes. As the degeneration process, called tropicalization, preserves many fundamental properties, tropical geometry provides important bridges and an exchange of methods between algebraic geometry, symplectic geometry and convex geometry; these links have been extremely fruitful and gave rise to remarkable results during the last 20 years. The main focus of the program will be on the most significant recent developments in tropical geometry and its applications. The following topics are particularly influential in the area and will be central in the program:
- real aspects of tropical geometry;
- tropical mirror symmetry and non-Archimedean geometry;
- tropical phenomena in symplectic geometry;
- matroids, combinatorial and algebraic aspects;
- tropical moduli spaces;
- tropical geometry and A1-homotopy theory.
Updated on Mar 13, 2024 02:34 PM PDT -
Program Inverse Problems and Applications
Organizers: Fioralba Cakoni (Rutgers University), Maarten de Hoop (Rice University), Anna Gilbert (Yale University), Katya Krupchyk (University of California, Irvine), Matti Lassas (University of Helsinki), LEAD Gunther Uhlmann (University of Washington)
Inverse problems (IP) arise in all fields of science and technology where a cause for an observed or desired effect is sought. In the last 50 years or so there has been substantial progress in the mathematical understanding of these problems but many questions remain open. The mathematics of these problems involves many areas in Mathematics including PDE, differential geometry, integral geometry, probability, statistics, complex analysis, numerical analysis, mathematical physics, data science, etc. Since the 2010 program at the then-Mathematical Sciences Research Institute (now Simons Laufer Mathematical Sciences Institute), there has been significant progress in inverse problems; many of the advances can be traced back to that program. However, there are still deep open questions remaining as well, some of which are discussed in this proposal. New research topics include the connection between IP and machine learning, IP for nonlinear equations, IP for nonlocal operators, and connections between statistics and IP.
Updated on Feb 28, 2024 03:01 PM PST
|
|
Upcoming Scientific Events |